REGISTRATION FEE
120€*
* Includes coffee breaks, guided tour and social dinner.
PHOTO GALLERY
SLIDES
Conference on Stochastic Analysis and Stochastic Partial Differential Equations
Sign into June 03, 2022
This event will be held on-site at the Centre de Recerca Matemàtica
* Wednesday sessions will take place at the Historical Building of the Universitat de Barcelona
INTRODUCTION
The goals of this conference are to bring together internationally renowned researchers working on various topics related to stochastic analysis and, particularly, to the theory and applications of stochastic partial differential equations, and to expose and attract graduate students and young researchers to this steadily growing area.
LIST OF SPEAKERS
Vlad Bally | Université Paris Est | Abstract
Dan Crisan | Imperial College London | Abstract
Anne de Bouard | École Polytechnique | Abstract
Arnaud Debussche | École Normale Supérieure de Rennes | Abstract
Aurélien Deya | Université de Lorraine | Abstract
Giulia di Nunno | University of Oslo | Abstract
Peter Friz | Technische Universität Berlin | Abstract
Archil Gulisashvili | Ohio University | Abstract
Istvan Gyongy | University of Edinburgh | Abstract
Peter Imkeller | Humboldt Universität Berlin | Abstract
Maria Jolis | Universitat Autònoma de Barcelona | Abstract
Arturo Kohatsu | Ritsumeikan University, Kyoto | Abstract
Sylvie Méléard | École Polytechnique | Abstract
Annie Millet | Université Paris 1 Pantheon-Sorbonne | Abstract
Carl Mueller | University of Rochester | Abstract
Ivan Nourdin | University of Luxembourg | Abstract
Giovanni Peccati | University of Luxembourg | Abstract
Michael Röckner | Universität Bielefeld | Abstract
Francesco Russo | Institut Polytechnique de Paris | Abstract
Samy Tindel | Purdue University | Abstract
Lorenzo Zambotti | Université de Paris | Abstract
CONTRIBUTED TALKS & POSTER PRESENTATIONS
To apply, please select the relevant option during the registration process.
SCIENTIFIC AND ORGANIZING COMMITTEE
Sandra Cerrai | University of Maryland
Mohammud Foondun | University of Strathclyde
Davar Khoshnevisan | University of Utah
David Márquez-Carreras | Universitat de Barcelona
Lluís Quer-Sardanyons | Universitat Autònoma de Barcelona
Josep Vives | Universitat de Barcelona
SCHEDULE (CEST/UTC+02:00)
Monday May 30th | Tuesday May 31st | Wednesday June 1st | Thursday June 2nd | Friday June 3rd | |
9:00 - 9:30 | Registration | Institutional Joan Guàrdia (rector Mgfc de la Universitat de Barcelona) Oriol Pujol (degà de la Facultat de Matemàtiques i Informàtica) Alícia Casals (presidenta de la Secció de Ciències i Tecnologia de l’Institut d’Estudis Catalans) Dolors Herbera i Espinal (presidenta de la Societat Catalana de Matemàtiques) Joaquim Ortega (director de l’IMUB) Victoria Otero (vicepresidenta de la Real Sociedad Matemática Española) Lluís Alsedà (director del Centre de Recerca Matemàtica) Volker Mehrmann (president de la European Mathematical Society) Marta Sanz-Solé (catedràtica de la Facultat de Matemàtiques i Informàtica) | |||
9:30 - 10:00 | Istvan Gyongy (University of Edinburgh) On nonlinear filtering of jump diffusions | Carl Mueller (University of Rochester) Self-Avoiding Models of Moving Polymers and Surfaces | Annie Millet (Université Paris 1 Pantheon-Sorbonne) Space-time discretization schemes for the 2D Navier Stokes equations with additive noise | Dan Crisan (Imperial College London) Solution properties of an incompressible Stochastic Euler system | |
10:00 - 10:15 | Coffee-Break | ||||
10:15 - 10:30 | Arturo Kohatsu-Higa (Ritsumeikan University) Probabilistic representation of the derivative of a one dimensional killed diffusion semigroup and associated BEL formula | Aurélien Deya (Université de Lorraine) A Young approach to hyperbolic Anderson model | Francesco Russo (Institut Polytechnique de Paris) Semimartingales with jumps, weak Dirichlet processes and path-dependent martingale problems | Arnaud Debussche (École Normale Supérieure de Rennes) Transport noise models from two-scale systems with additive noise in fluid dynamics | |
10:30 - 11:00 | UB Colloquiums Michael Röckner (Universität Bielefeld) Taming Uncertainty and Profiting from Randomness | ||||
11:00 - 11:30 | Coffee-Break | Coffee-Break | Coffee-Break | Coffee-Break | |
11:30 - 12:00 | Giulia Di Nunno (University of Oslo) Optimal control of Volterra type equations: from finite to infinite dimensions and return | Ivan Nourdin (University of Luxembourg) The Breuer-Major theorem: old and new | Coffee-Break | Anne de Bouard (École Polytechnique) Stochastic Gross-Pitaevskii equation and invariant measure | Vlad Bally (Université Paris Est) Construction of Boltzmann and McKean Vlasov Type Flows (The Sewing Lemma Approach) |
12:00 -12:15 | UB Colloquiums Samy Tindel (Purdue University) Waving Marta Goodbye | ||||
12:15 - 13:00 | Sylvie Méléard (École Polytechnique) Filling the gap between individual-based evolutionary models and Hamilton-Jacobi equations | Giovanni Peccati (University of Luxembourg) Wiener chaos and the geometry of Gaussian random waves | Peter Friz (Technische Universität Berlin) Rough stochastic Analysis | Maria Jolis (Universitat Autònoma de Barcelona) Some results of convergence in law to the solution of stochastic PDEs | |
13:00 - 13:15 | LUNCH | LUNCH | Closing | LUNCH | LUNCH |
13:00 - 15:00 | |||||
15:00 - 15:45 | Archil Gulisashvili (Ohio University) Multivariate Stochastic Volatility Models and Large Deviation Principles | Peter Imkeller (Humboldt Universität Berlin) Geometric properties of some rough Weierstrass and Takagi type curves: SBR measure and local time | Lorenzo Zambotti (Université de Paris) Renormalisation from Quantum Field Theory to SPDEs | ||
15:45 - 16:15 | Sonja Cox (University of Amsterdam) An affine infinite-dimensional stochastic volatility model | Marco Rehmeier (Bielefeld University) Nonuniqueness in law for stochastic hypodissipative Navier-Stokes equations | Oana Lang (Imperial College London) On maximal and global solutions for stochastic shallow water models | ||
16:15 - 16:45 | Coffee-Break | Coffee-Break | Coffee-Break | ||
16:45 - 17:00 | Adrián Hinojosa Calleja (Universitat de Barcelona) A linear stochastic biharmonic heat equation: hitting probabilities | Adrian Falkowski (Nicolaus Copernicus University in Toru) Mean reflected stochastic differential equations with two constraints | Liliana Peralta (Universidad Nacional Autónoma de México) Controllability of some semilinear parabolic SPDEs | ||
17:00 - 17:15 | Guided visit to Sagrada Família | ||||
17:15 - 17:45 | Vitalii Makogin (University of Ulm) Fractional integral equations with weighted Takagi-Landsberg functions | Asma Khedher (University of Amsterdam) Utility maximisation and change of variable formulas for time-changed dynamics | Carsten Chong (Columbia University) The stochastic heat equation with multiplicative Lévy noise: Existence, intermittency, and multifractality | ||
17:45 - 18:30 | Poster Session | ||||
18:30 - 19:30 | |||||
20:00 | Dinner in Barcelona |
contributed talks
poster session
acknowledgements
REGISTRATION
INVOICE/PAYMENT INFORMATION
IF YOUR INSTITUTION COVERS YOUR REGISTRATION FEE: Please note that, in case your institution is paying for the registration via bank transfer, you will have to indicate your institution details and choose “Transfer” as the payment method at the end of the process.
UPF | UB | UPC | UAB
*If the paying institution is the UPF / UB/ UPC / UAB, after registering, please send an email to vramirez@crm.cat with your name and the institution internal reference number that we will need to issue the electronic invoice. Please, send us the Project code covering the registration if needed.
Paying by credit card
IF YOU PAY VIA CREDIT CARD but you need to provide the invoice to your institution to be reimbursed, please note that we will also need you to send an email to vramirez@crm.cat providing the internal reference number given by your institution and the code of the Project covering the registration (if necessary).
List of participants
| Name | Institution |
|---|---|
| Stefane Saize | Universidade Eduardo Mondlane |
| Adrián Hinojosa Calleja | Unnafiliated |
| Annie Millet | University Paris 1 Panthéon Sorbonne |
| Ivan Nourdin | University of Luxembourg |
| Arnaud Debussche | Ecole normale supérieure de Rennes |
| Wanchang Zhang | The Technical University of Catalonia |
| Víctor Hernández-Santamaría | Universidad Nacional Autónoma de México |
| Varun Chhabra | Defence Science Technology Laboratory |
| Giulia Di Nunno | University of Oslo |
| David Marquez-Carreras | Universitat de Barcelona |
| José M. Corcuera | Universitat de Barcelona |
| Marta Sanz-Solé | Universitat de Barcelona |
| Xavier Bardina | Universitat Autònoma de Barcelona |
| Giulia Binotto | Universitat Autònoma de Barcelona |
| Aureli Alabert | Universitat Autònoma de Barcelona |
| Alejandra Cabaña | Universitat Autònoma de Barcelona |
| Maria Jolis | Universitat Autònoma de Barcelona |
| Elisa Alòs | Universitat Pompeu Fabra |
| Eulalia Nualart | Universitat Pompeu Fabra |
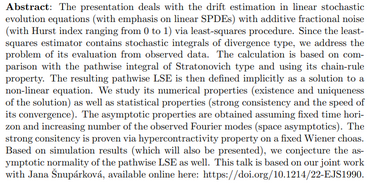
| Jana Šnupárková | University of Chemistry and Technology |
| Pavel Kríž | Charles University in Prague |
| Ondrej Tybl | Charles University in Prague |
| Anne de Bouard | École Polytechnique |
| Sylvie Meleard | École Polytechnique |
| Lorenzo Zambotti | Pierre-and-Marie-Curie University |
| Francesco Russo | University of Paris 13 |
| Vitalii Makogin | University of Ulm |
| Marco Rehmeier | Bielefeld University |
| Röckner Michael | Bielefeld University |
| Lena Schadow | University of Lübeck |
| Felix Kastner | University of Lübeck |
| Liliana Peralta | National Autonomous University of Mexico |
| Asma Khedher | University of Amsterdam |
| Sonja Cox | University of Amsterdam |
| Max Sauerbrey | Delft University of Technology |
| Adrian Falkowski | Nicolaus Copernicus University in Toru? |
| Neeraj Bhauryal | University of Lisbon |
| Qiao Huang | University of Lisbon |
| Ana Merkle | University of Belgrade |
| Matija Vidmar | University of Ljubljana |
| Dan Crisan | Imperial College London |
| Daniel Goodair | Imperial College London |
| Oana Lang | Imperial College London |
| Fabian Germ | University of Edinburgh |
| Gergely Bodó | King's College London |
| Stefano Bruno | University of Bath |
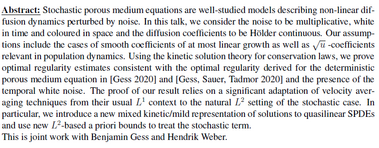
| Mohammud Foondun | University of Strathclyde |
| Carsten Chong | Columbia University |
| Carl Mueller | University of Rochester |
| Yukun Li | University of Central Florida |
| Archil Gulisashvili | Ohio University |
lodging information
ON-CAMPUS AND BELLATERRA
BARCELONA AND OFF-CAMPUS
|
For inquiries about this event please contact the research programs coordinator Ms. Núria Hernández at nhernandez@crm.cat
|
A theory of stochastic geometric mechanics
Qiao Huang | University of Lisbon
Classical geometric mechanics, including symmetries, Lagrangian and Hamiltonian mechanics, and the Hamilton-Jacobi theory, are based on classical geometric structures such as jets, symplectic structures and contact structures. In this poster, we will continue the forgotten framework of the second-order (or stochastic) differential geometry developed originally by L. Schwartz and P.-A. Meyer, to construct second-order (or stochastic) counterparts of those classical structures. Based on these, we then study symmetries of SDEs, stochastic Lagrangian and Hamiltonian mechanics as well as their connections with the second-order Hamilton-Jacobi-Bellman equation. More precisely, stochastic prolongation formulae are established and symmetries of SDEs and mixed-order Cartan symmetries are studied. Stochastic Hamilton’s equations are formulated via Nelson’s mean derivatives. Canonical transformations for mixed-order contact structure lead to the second-order Hamilton-Jacobi-Bellman equation. When a stochastic variational problem is set up, a stochastic Euler-Lagrange equation is derived and its equivalence with a reduction of stochastic Hamilton’s equations and a Hamilton-Jacobi-Bellman equation are proved. The latter equivalence suggests an alternative formulation to the Schr\”odinger problem equivalent to the viewpoint of optimal transport. A stochastic Noether’s theorem is also established where the stochastic conserved quantities are martingales. This poster is based on joint work with J.-C. Zambrini.
Controllability of some semilinear parabolic SPDEs
Liliana Peralta | Universidad Nacional Autónoma de México
In this talk, we will present some controllability results for stochastic parabolic equations. In particular, we shall present a new controllability result for a stochastic parabolic equation with globally Lipschitz nonlinearities in the drift and the diffusion terms. For this, we propose a new twist on a classical strategy for controlling linear parabolic equations which allows us to circumvent the well-known lack of compactness embedding for the solution spaces arising in the study of controllability problems for stochastic PDEs.
On maximal and global solutions for stochastic shallow water models
In this talk I will present the analytical properties of a stochastic shallow water model which has been derived using the Location Uncertainty (Mémin, 2014) approach. We prove that there exists a unique maximal strong solution and a unique global weak solution, using a method which relies on a Cauchy approximating sequence. Our method is very convenient as although the strong solution exists in a higher order Sobolev space, we show that it suffices to prove the Cauchy property in L^2. This is different from the approaches currently used in the literature which rely mainly on tightness arguments.
A linear stochastic biharmonic heat equation: hitting probabilities
We find hitting probabilities estimations for the solution of a linear stochastic biharmonic biharmonic heat equation driven by white noise. We first study the anisotropies of the canonical metric of the solution, in particular when the spatial dimension d=2, they include a z(-log(z))^(1/2) term. Applying the criteria proved in previous works, we establish upper and lower bounds for the probabilities that the path process hits bounded Borel sets. This yield results on the polarity of sets and on the Hausdorff dimension of the path process.
This is joint work with M. Sanz-Solé.
Utility maximisation and change of variable formulas for time-changed dynamics
In this paper we derive novel change of variable formulas for stochastic integrals w.r.t. a time-changed Brownian motion where we assume that the time change is an increasing stochastic process with finitely many jumps in a bounded set of the positive half-line and is independent of the Brownian motion. As an application we consider the problem of maximising the expected utility of the terminal wealth in a semimartingale setting, where the semimartin-gale is written in terms of a time-changed Brownian motion and a finite variation process. To solve this problem, we use an initial enlargement of filtration and our change of variable formulas to shift the problem to a maximisation problem under the enlarged filtration for models driven by a Brownian motion and a finite variation process. The latter problem can be solved by using martingale properties. Then applying again the change of variable formula, we derive the optimal strategy for the original problem for a power utility and for a logarithmic utility. This is based on a joint work with Giulia Di Nunno, Hannes Haferkorn, and Michèle Van-
maele.
The stochastic heat equation with multiplicative Lévy noise: Existence, intermittency, and multifractality
Carsten Chong | Columbia University
In this talk, we consider the stochastic heat equation driven by a multiplicative Léevy noise in arbitrary dimension d >= 1. We prove the existence of solutions under an optimal condition if d = 1; 2 and a close-to-optimal condition if d >= 3. Under an assumption that is general enough to include stable noises, we further prove that the solution is unique. Furthermore, for any p > 0, we derive upper and lower bounds on the moment Lyapunovexponents of order p of the solution. One of our most striking findings is that the solution to the SHE is strongly intermittent for any non-trivial Lévy measure, at any disorder intensity, in any dimension d >= 1. The absence of a phase transition from a non-intermittent to an intermittent regime in d >= 3 is in sharp contrast with the SHE on the lattice or the SHE with Gaussian noise. If time permits, I will further discuss the multifractal behavior of intermittency islands in snapshots of the solution. This talk is based on joint works with Quentin Berger, Hubert Lacoin and Péter Kevei.
An affine infinite-dimensional stochastic volatility model
Sonja Cox| University of Amsterdam
I will first briefly explain what an affine stochastic process is. Such processes have received a considerable amount of attention in the past years due to their tractability and (relative) flexibility For example, in 2011 Cuchiero, Filipovic, Mayerhofer, and Teichmann provided a characterization of all affine processes taking values in the cone of non-negative semi-definite matrices. The motivation for doing so was to construct tractable finite-dimenstional stochastic volatility models.
Our goal was to obtain similar infinite-dimensional stochastic volatility models. To this end, we established existence of affine processes in the cone of positive self-adjoint Hilbert-Schmidt operators. In my talk, I will discuss the details of our result, the challenges of the infinite-dimensional setting, and some open questions.
Self-Avoiding Models of Moving Polymers and Surfaces
Carl Mueller | University of Rochester (This is joint work with Eyal Neuman)
Polymer models give rise to some of the most challenging problems inprobability and statistical physics. We typically model a polymer using a random walk, where the time parameter n of the walk represents distance along the polymer starting from one end. That is, we imagine that the polymer is built up by adding molecules one by one at random angles. We usually include a self-avoidance term, re ecting the idea that different parts of the polymer cannot be in the same place at the same time. A diffcult problem, unsolved in the most important physical cases, is to predict the end-to-end distance or radius of the polymer.
In this talk, I will discuss two extensions of the random polymer model.
1. Moving polymers can be modeled by stochastic partial differential equations. If the polymer takes values in one-dimensional Euclidean space, we give fairly sharp upper and lower bounds for its radius. We find that there is more stretching than in typical one-dimensional polymer models that do not have time dependence.
2. Random surfaces can be modeled by elastic manifolds, also called discrete Gaussian free fields. These models originate in quantum field theory. If the dimensions of the parameter space and the range are the same, we can derive bounds on the radius of the polymer. These bounds are fairly sharp in two dimensions.
We will explain the models mentioned above and give an outline of our proof techniques.
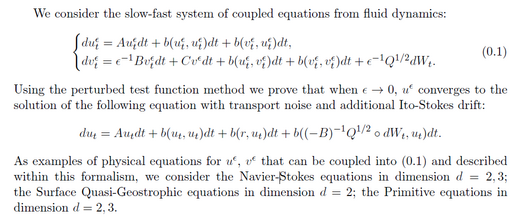
Transport noise models from two-scale systems with additive noise in fluid dynamics
Arnaud Debussche | École Normale Supérieure de Rennes
Taming Uncertainty and Profiting from Randomness
Michael Röckner | Universität Bielefeld
The title of this talk is a short form of the title of a major research project (a socalled Collaborative Research Centre, funded by the German Research Foundation, DFG), currently running at Bielefeld University. It is based on the claim that randomness, which is ubiquitous and cannot be avoided, neither in the natural sciences, nor in the humanities, has in fact two “faces“, one is a kind of a nuisance, which destroys one’s observations or measurements, is hard to quantify and causes uncertainty about the correctness of one’s final conclusions. So, one has to look for means and to develop tools for “taming“ it. On the other hand, in many cases randomness can help to understand observed phenomena which otherwise would remain a mystery, and in addition its mathematical analysis may lead to a deeper understanding of the underlying mechanisms, thus leading to resolving seemingly contradictory conclusions, which with no doubt is a valuable “profit“. In the talk I shall present some examples for the above claim and try to explain what mathematics, in particular stochastic analysis, offers for both “taming uncertainty“ and “profiting from randomness“. One eminent instance for both the latter will be the mathematical approachto understanding dynamical processes under the influence of “noise“, which appear in all sciences. The technical term of this mathematical approach is “stochastic partial differential equations“, but despite this for non-mathematicians maybe a bit “terrifying“ name, I think these can be understood quite well on an intuitive level from a certain view point, as I shall try to explain in the talk.
Optimal control of Volterra type equations: from finite to infinite dimensions and return
Giulia di Nunno | University of Oslo
We consider a stochastic optimal control problem for Volterra type forward dynamics, which are typically non-Markovian in nature. A direct application of dynamic programming is not feasible. So we propose to lift the problem to infinite dimensions, where we can recover Markovianity. For this we work with Banach-valued processes. In this framework we tackle the optimal control problem by studying the corresponding Hamilton-Jacobi-Bellman equation, which can be formulated thanks to the appropriate lift. Finally, we reconnect the solution of the infinite dimensional problem to the original finite dimensional one.
Rough stochastic Analysis
Peter Friz | TU and WIAS Berlin
We build a hybrid theory which seamlessly combines classical stochastic – and rough analytic considerations. In particular, we give, for the first time, direct meaning to rough Ito diffusions driven simultaneously by a Brownian motion B (in Ito sense) and a rough path W (in Lyons’ sense). This can be viewed as partial robustification of SDEs with two Brownian noise components, typical in filtering situations. In presence of some Markovian structure, we are led to classes of rough/stochastic partial differential equations, including non-linear ones of HJB or mean-field type. Compared with previous approaches based on flow-transformation this approach offers an intrinsic understanding of the underlying process, well-adapted to discrete approximations and with minimal regularity demands. Propagation of chaos for particle systems with common noise is another application of our theory.
Joint work with K. Le (TU Berlin) and A. Hocquet (TU Berlin).
Waving Marta Goodbye
Samy Tindel | Purdue University
In this talk I will first give a brief overview of some standard stochastic PDE models. Then I will describe some of Marta’s fundamental contributions to the theory of stochastic wave equations. This includes challenging problems such as the definition of noisy wave equations in higher dimensions, as well as further properties of the solution. Those results are based on nontrivial extensions of Itô type integrals and advanced Malliavin calculus tools, for which I intend to give a short and non technical introduction. Eventually I will give an account on more recent pathwise approaches to noisy wave equations, which are still the object of active research.
Space-time discretization schemes for the 2D Navier Stokes equations with additive noise
Annie Millet | Université Paris 1, SAMM (and LPSM)

Multivariate Stochastic Volatility Models and Large Deviation Principles
Archil Gulisashvili | Ohio University
We establish a comprehensive sample path large deviation principle (LDP) for log-processes associated with multivariate time-inhomogeneous stochastic volatility models. Examples of models for which the new LDP holds include Gaussian models, non-Gaussian fractional models, mixed models, models with reflection, and models in which the volatility process is a solution to a Volterra type stochastic integral equation. The LDP for log-processes is used to obtain large deviation style asymptotic formulas for the distribution function of the first exit time of a log-process from an open set and for the price of a multidimensional binary barrier option. We also prove a sample path LDP for solutions to Volterra type stochastic integral equations with predictable coefficients depending on auxiliary stochastic processes.
Forward integration of bounded variation
coefficients with respect to Hölder continuous processes
Jorge A. León | Cinvestav-IPN
In this talk, we study the forward integral with respect to H older continuous stochastic processes Y with exponent bigger than 1/2 in the Russo and Vallois sense. Here, the integrands have the form f(Y), where f is a function of bounded variation. We show that this integral agrees with the generalized Stieltjes integral given by Zähle and that, in the case that Y is fractional Brownian motion, this forward integral is equal to the divergence operator plus a trace term, which is related to the local time of Y.
Filling the gap between individual-based evolutionary models and Hamilton-Jacobi equations
Sylvie Méléard | École Polytechnique
We consider a stochastic model for the evolution of a discrete population structured by a trait taking finitely many values on a grid of [0, 1], with mutation and selection. Traits are vertically inherited unless a mutation occurs, and influences the birth and death rates. We focus on a parameter scaling where individual mutations are small but not rare, and where the grid mesh for the trait values becomes smaller and smaller. When considering the evolution of the population in long time scales, the contribution of small sub-population is important. Our main result quantifies the asymptotic dynamics of subpopulation sizes on a logarithmic scale. We establish (and this is the first proof of this kind in the literature to our knowledge) that under our rescaling, the stochastic discrete process converges to the viscosity solution of a Hamilton-Jacobi equation. It is a joint work with N. Champagnat, S. Mirrahimi and C.V. Tran.

On nonlinear filtering of jump diffusions
Istvan Gyongy | University of Edinburgh
Partially observed jump diffusions, described by stochastic differential equations driven by Wiener processes and Poisson measures are considered. In the first part of the talk the filtering equations, i.e., the equations for conditional distribution P(t,dx) and for the unnormalised conditional distribution of the unobserved component at time t, given the observations until t, are presented.
These are (possibly) degenerate stochastic integro-differential equations. By the help of these equations new results on the existence and on the regularity properties of the density functions P(t,dx)/dx are established.
The talk is based on joint papers with Fabian Germ from Edinburgh University.
A probabilistic representation of the derivative of a
one dimensional killed diffusion semigroup and associated BEL formula
Arturo Kohatsu-Higa | Department of Mathematical Sciences, Ritsumeikan University 1-1-1
Nojihigashi, Kusatsu, Shiga, 525-8577, Japan.
We provide a probabilistic representation for the derivative of the semigroup corresponding to a diffusion process killed at the boundary of a half interval. In particular, we show that the derivative of the semi-group can be expressed as the expected value of a functional of a reflected diffusion process. Furthermore, as an application, we obtain a Bismut-Elworthy-Li formula.
This is joint work with Dan Crisan.
Some results of convergence in law to the solution of stochastic PDEs.
Maria Jolis | Universitat Autònoma de Barcelona
We study PDEs perturbed by some types of random noises.
These noises approximate in some sense either the Gaussian white noise or the Gaussian noise white in time and fractional in space. We will present several results of convergence in law of the solutions of
our PDEs to the corresponding solutions of the stochastic PDEs.
These kind of results justify the use of the stochastic PDEs with the Gaussian noises as good models in situations where the noises have more complicated law but are “near” the Gaussian ones.
Stochastic Gross-Pitaevskii equation and invariant measure
Anne de Bouard | CMAP, CNRS, Ecole Polytechnique, Palaiseau, France
In the last twenty years, mean field models taking account of thermal fluctuations, aiming to describe Bose-Einstein condensates close to critical condensation temperature have been introduced, the so called “Stochastic Projected Gross Pitaevskii equation”. We will describe some mathematical results about the analysis and long-time dynamics of a non-truncated version of the model, a stochastic PDE which is a complex Ginzburg Landau equation with a confining harmonic potential and additive space-time white noise. We will discuss in particular the analysis of the model in the two-dimensional space situation, in which renormalization is necessary in order to make sense of the solutions, and the study of the formal Gibbs measure presents challenging issues.
This is based on joint works with A. Debussche (ENS Rennes, France) and R. Fukuizumi (Tohoku University, Japan)
Wiener chaos and the geometry of Gaussian random waves
Giovanni Peccati | University of Luxembourg
I will present an overview of a recent stream of research – focussing on the geometric study of Gaussian random waves (that is, Gaussian Laplace eigenfunctions) by using tools of Gaussian analysis – in particular, Wiener chaos and Breuer-Major type results. If time permits, I will discuss some challenging open problems, related e.g. to functional convergence and the optimal coupling of Gaussian fields.
Renormalisation from Quantum Field Theory to SPDEs
Lorenzo Zambotti | Université de Paris
In the last decade there has been a lot of work on singular SPDEs which require a renormalisation, namely a procedure of regularisation and « correction » since it is necessary to « remove » some diverging constants or more generally polynomial terms. This rather mysterious phenomenon is well-known in Quantum Field Theory where it is related to ultra-violet divergences. In this talk I will try to compare to the approaches, showing that they are similarities but also important differences, such that it is still broadly impossible to translate the results of one theory in the context of the other.
Construction of Boltzmann and McKean Vlasov Type Flows (The Sewing Lemma Approach)
Vlad Bally | Université Paris Est
We are concerned with a mixture of Boltzmann and McKean-Vlasov type equations, this means (in probabilistic terms) equations with coefficients depending on the law of the solution itself, and driven by a Poisson point measure with the intensity depending also on the law of the solution. Both the analytical Boltzmann equation and the probabilistic interpretation initiated by Tanaka [33, 34] have intensively been discussed in the literature for specific models related to the behavior of gas molecules. In this paper, we consider general abstract coefficients that may include mean field effects and then we discuss the link with specific models as well. In contrast with the usual approach in which integral equations are used in order to state the problem, we employ here a new formulation of the problem in terms of flows of endomorphisms on the space of probability measure endowed with the Wasserstein distance. This point of view already appeared in the framework of rough differential equations. Our results concern existence and uniqueness of the solution, in the formulation of flows, but we also prove that the ”flow solution” is a solution of the classical integral weak equation and admits a probabilistic interpretation. Moreover, we obtain stability results and regularity with respect to the time for such solutions. Finally we prove the convergence of empirical measures based on particle systems to the solution of our problem, and we obtain the rate of convergence. We discuss as examples the homogeneous and the inhomogeneous Boltzmann (Enskog) equation with hard potentials.
Geometric properties of some rough Weierstrass and Takagi type curves: SBR measure and local time
Peter Imkeller | Humboldt Universität Berlin
We investigate geometric properties of graphs ofWeierstrass or Tak- agi type functions, represented by series based on smooth functions. They are Holder continuous, and can be embedded into smooth dy- namical systems, where their graphs emerge as pullback attractors. It turns out that occupation measures and Sinai-Bowen-Ruelle (SBR) measures on their stable manifolds are dual by time reversal. A suit- able version of approximate self similarity for deterministic functions allows to “telescope” small scale properties from macroscopic ones. As a consequence, absolute continuity of the SBR measure is seen to be dual to the existence of local time. The link between the rough curves considered and smooth dynamical systems can be generalized in various ways. Applications to regularization of singular ODE by rough signals are on our agenda. This is joint work with O. Pamen (U Liverpool and AIMS Ghana) and G. dos Reis (U Edinburgh).
Solution properties of an incompressible Stochastic Euler system
Dan Crisan | Imperial College London
I will present some results for well-posedness of the 3D and 2D Euler equation for the incompressible flow of an ideal fluid perturbed by an additional stochastic divergence-free, Lie-advecting vector field. In 3D, the equation is locally well-posed in regular spaces. A Beale–Kato–Majda type criterion charactecterizes the blow-up time. In 2D, the eqaution has a unique global strong solution and the initial smoothness of the solution is preserved. If there is time I will present a rough path version of the model.
This is joint work with Oana Lang, Franco Flandoli and Darryl Holm and is based on the papers:
[1] O Lang, D Crisan, Well-posedness for a stochastic 2D Euler equation with transport noise, Stochastics and Partial Differential Equations: Analysis and Computations, 1-48, 2022.
[2] D. Crisan, F Flandoli, DD Holm, Solution properties of a 3D stochastic Euler fluid equation, Journal of Nonlinear Science 29 (3), 813-870, 2019.